
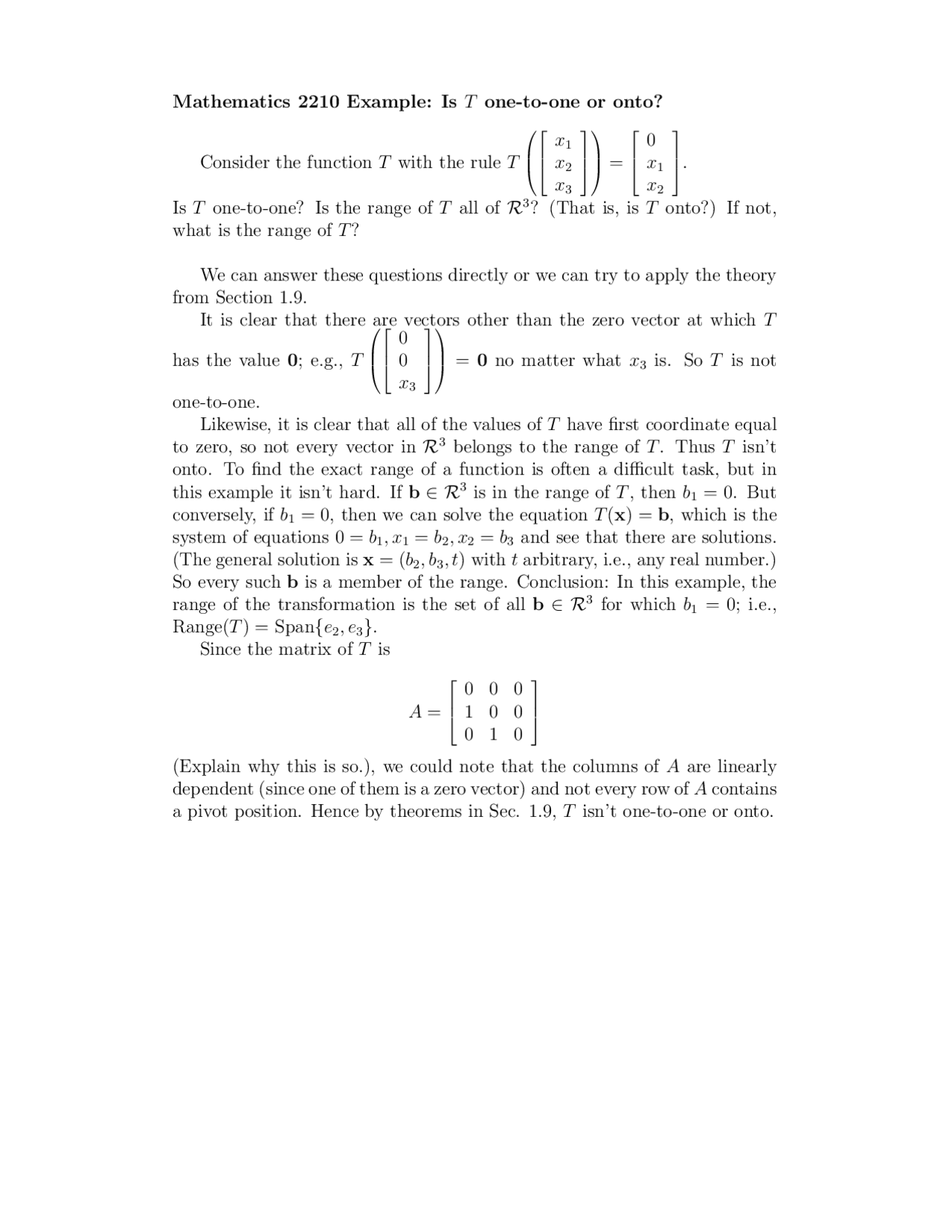
Therefore, f: A → B is ansurjective function. Here, the entire element on B has a domain element on A or we can say element 1 and 8 & 5 and 9 has same range 2 & 4 respectively. Solution: From the question itself we get, When we compose onto functions, the result will be onto function only.Įxample: Let A=. Every onto function has a right inverse and every function with a right inverse is an onto function.Because of this it’s not an onto function In this section, we define these concepts 'officially'' in terms of preimages, and explore some easy examples and consequences. If a function does not map two different elements in the domain to the same element in the range, it is one-to-one or injective. In the second figure it can be seen that one element in Set B is not mapped with any of the element of set A. If the codomain of a function is also its range, then the function is onto or surjective. You can see in the figure that for each element of B there is a pre-image or a matching element in Set A. Ifthere is at least one or more than one element matching with A for every element of B, then the function is said to be onto function or surjective function. This difference is hard to see in the examples you have asked about, because the verb, put, itself, suggests moving something from one place to another. To explain Onto function consider two sets, Set A and Set B which consist of elements. Therefore, the given function f is one-one. (a 1 2 + a 1a 2 + a 2 2) = 0 is not considered because there are no real values of a 1 and a 2. In the same manner if x ≠ y, then f(x) ≠ f(y)įormally, it is stated as, if f(x)=f(y) implies x=y, then f is one-to-one mapped or f is 1-1.Įxample 1: Show that f: R → R defined as f(a) = 3a 3 – 4 is one to one function? The function is said to be one to one if for all x and y in A, To understand this, let us consider ‘f’ is a function whose domain is set A. One-to-One functions define that each element of one set say Set (A) is mapped with a unique element of another set, say, Set (B). One-to-one function or injective function is one of the most common functions used.


 0 kommentar(er)
0 kommentar(er)
